How to save Fourier series approximated signal to a WAV file

Question

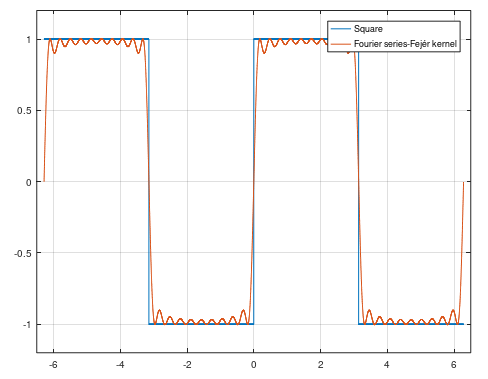
I changed this Matlab/Octave code to approximate square wave by using combination of Fourier series and Fejér taper:

    % needed for Octave ------------------------------    
    pkg load signal
    % ------------------------------------------------
    
    % Stage 1 ----------------------------------------
    figure
    N=20;                               % harmonics
    fs = 44100;
    fc = 440;
    w = 2 * pi * fc;
    r_start=0;
    r_end=10;
    r_step=1/fs;
    t = r_start:r_step:r_end;           % range
    w_sqr = square(w*t);          % Square wave [-1:1]
    
    plot(t,w_sqr);
    axis([r_start r_end -1.2 1.2]);
    grid on;
    hold on;
    audiowrite("squarewave.vaw", w_sqr, fs);
    
    % Approximation: Combined 
    % Fourier series-Fejér taper (Tukey window?) -----------
    %i=1;
    %sum=0;
    %r=t;
    %for t=r
    %  for n=1:N
    %    %sum = sum + (2*sin(n*t)+sin(pi*n-n*t)-sin(n*t+pi*n))/(2*pi*n) % Fourier series
    %    %sum = sum + ((1-n/(N+1))*(-1)*sin(n*t)*(cos(n*pi)-1))/(n*pi); % Fejér taper
    %    sum = sum + ((n-2*N-2)*(cos(pi*n)-1)*sin(n*w*t))/(2*pi*n*(N + 1)); % (Fourier+Fejér)/2
    %  end
    %   Ffej(i)= sign(sum)*(1/2) + sum;
    %  i=i+1;
    %  sum=0;
    %end;
    %Ffej=Ffej';

    sum_ = sum((((1:N)-2*N-2).*(cos(pi*(1:N))-1).*sin((1:N).*w.*(0:1/fs:1)'))./(2*pi*(1:N)*(N + 1)), 2); 
    Ffej = 2*sum_;
    Ffej = Ffej';

    plot(t,Ffej);
    axis([r_start r_end -1.2 1.2]);
    legend('Square', 'Fourier series-Fejér taper');
    
    audiowrite("approximated_squarewave.wav, Ffej, fs);

which results this plot:

I would like to save these signals to .wav files by using Matlab/Octave audiowrite() command.

What changes are needed to be done in code to save an approximation of 440Hz square wave sound sampled at 44100 Hz? My thought is that range t must be changed to support sample rate range and frequency but, how is the situation with calculation of Ffej ... or is data of Ffej directly usable in audiowrite() command?

Answer 1

OK, after digging the case by myself I managed to generate square wave files for both cases. Updated the source code found in my opening post.

In case of combined Fourier Series and Fejér taper approximations of square wave signal, result looks like this (plot done with Audacity):

and spectrum like this (Plot done with Octave):

(green = w_sqr, black dotted = Ffej, fc=1230Hz, fs=44100Hz, w_sqr and Ffej are data holders from source code)