I am really trying to digest this paper, but the more I read it again, the more I realize that I never fully understood it.
Basically, I am trying to get an example where I can check the similarities between (28) and (29) to see I am doing things properly.
Here are some important parts that I am try to follow:
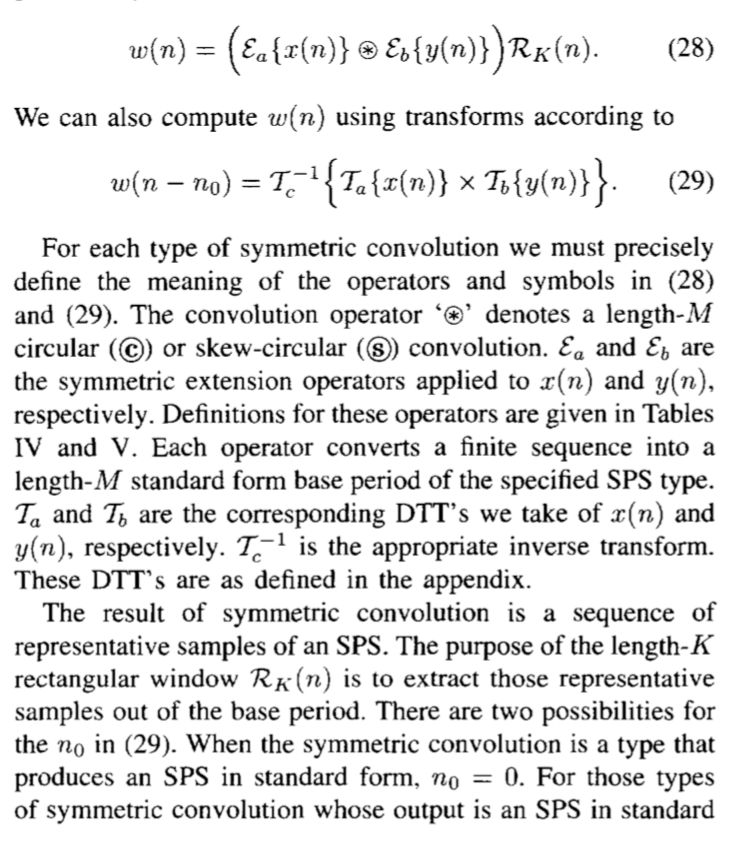
- Eq 28 and Eq 29
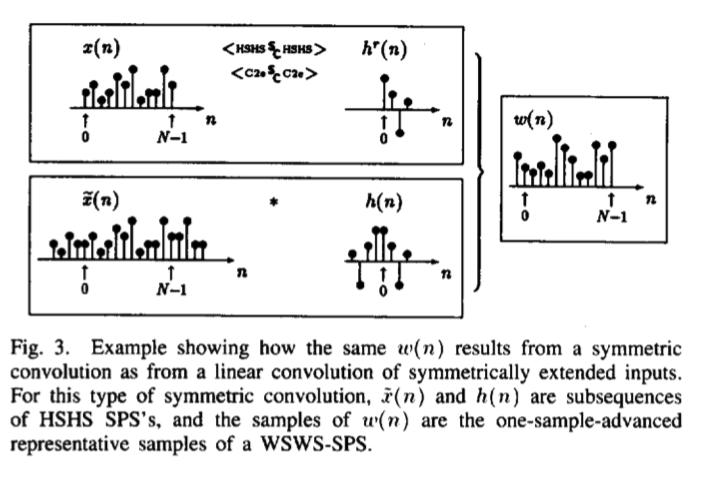
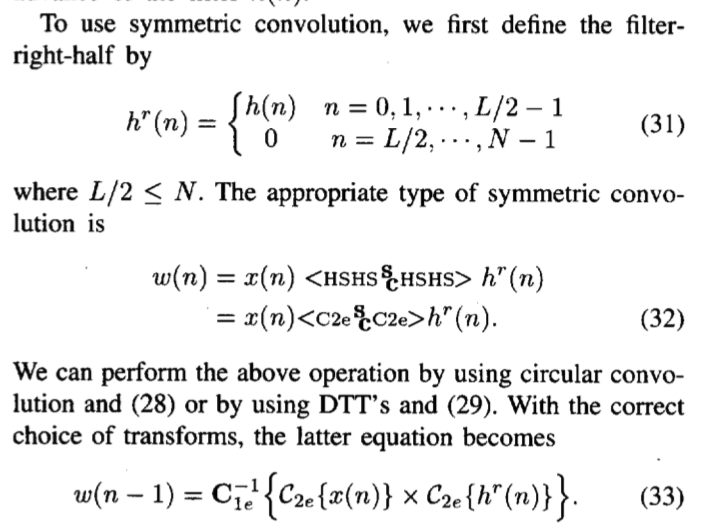
- I am using DCT-II as $T_a$ and $T_b$ and iDCT-I as $T_c$. Thus according to the paper, I must use the extensions HSHS on both $x$ and $y$, them the output will be a circular convolution of their extended versions
- Then, we should be able to match this output by applying a multiplication operations on DCT representation of their unextended versions.
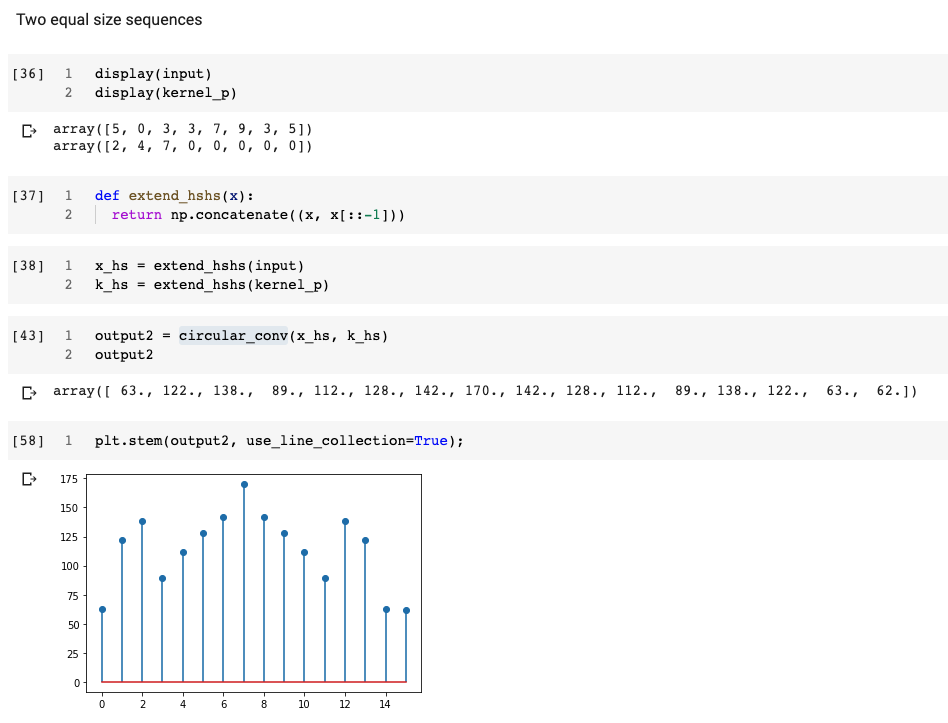
- However, when I get to reproduce this, I don't see this resemblance.
- There is no window $\mathcal{R}(n)$ I could extract from the first output bellow, that would match the second one.
- It could be that the output values using the transforms would needed to be scaled, somehow, but even doing so, it would not change their shape in the plot, which I am using to compare both outputs.
- According to the paper, I should get an extra sample on the approach using the transforms, since it outputs on the interval [-1, N-1], I am also not sure where that comes from.
In short: How to properly interpret and demonstrate this correspondence between (28) and (29) this combination of $T_a = T_b = \text{DCT-II}$ and $T_c = \text{DCT-I}$?