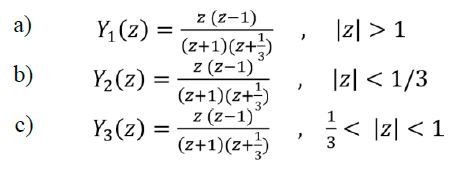What is the causality & stability status for three cases shown (aso in attached photo) ?
$$H(z) = \frac{z(z-1)}{(z+1)(z+\frac{1}{3})} $$
for three possible regions of convergence as:
a-) |z| > 1
b-) |z| < 1/3
c-) 1/3 < |z| < 1
Am i ok as below?
a) Causal & marginally stable
b) anti-causal & unstable
c) non-causal & marginally stable
Yes, all answers given by you are fine.
Causal: If ROC is outside the outermost pole
Left sided: If ROC is inside the innermost pole
Stable: If ROC contains the unit circle (marginally stable if it touches unit circle)
I will only give you hints 1. Casual if |Z|>|a| 2. Stable if Roc contains unit circle So non causal if |Z|<|a|, unstable if Roc don't contain unit circle & marginally stable if poles are on unit circle.
