Reading the amplitude values of a FFT in ImageJ

Question

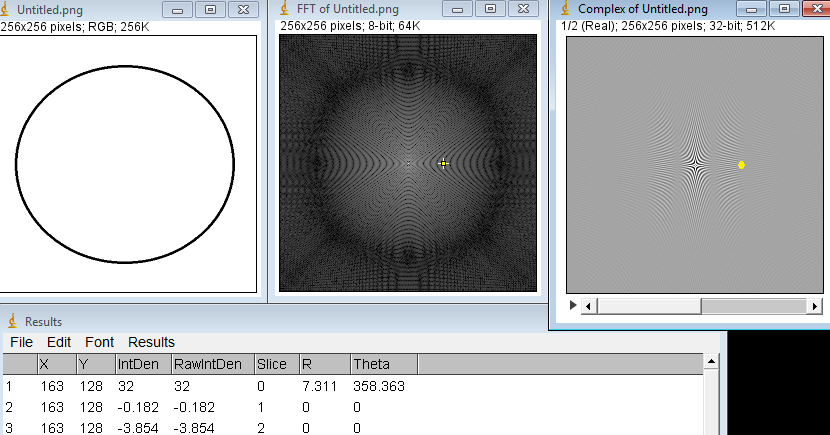
Readings the output of the FFT of the picture of a circle in ImageJ at some random point $(163,128)$ I get $X_{\text{Re}}=-0.182$ and $X_{\text{Im}}=-3.854$, using the Complex plot (yellow dot). The value on the output on the FFT plot is $32$ (cross cursor symbol):

I don't understand why the relation between this latter value ($32$), which I presumed it was the amplitude, and the real and imaginary components, i.e. $\vert X[k]\vert=\sqrt{X_\text{Re}^2+X_\text{Im}^2}$, is not fulfilled.

I had come across the power spectrum logarithmic quote in the ImageJ manual:

The frequency domain image is stored as 32-bit float FHT 
attached to the 8-bit image that displays the power spectrum.

but I can't find a formula to translate the values between the output on ImageJ and the square root. For instance $\log 32 =3.466$, while $\sqrt{(-0.182)^2 + (-3.854)}=3.858$. I wonder if there is some normalization across the image, for example to explain this difference.

This is likely related to the Hartley transform used in ImageJ (page 39).

Answer 1

Looking at the source code, the power spectrum image is using a logarithmic scale, adjusted based on the image's dynamic range such that the minimum value (not less than 1) gives a value of 1 and the maximum value gives a value of 254:

if (min<1.0)
    min = 0f;
else
min = (float)Math.log(min);
max = (float)Math.log(max);
scale = (float)(253.0/(max-min));

...
if (r<1f)
    r = 0f;
else
r = (float)Math.log(r);
ps[base+col] = (byte)(((r-min)*scale+0.5)+1);

Making a broad assumption that at least one of those darker pixel is a null would imply that the computed min is close to 0. With this assumption we can proceed to find max as follows:

$$ \begin{align} 32 &= \left\lfloor \ln(3.858) \frac{253}{\ln(r_\max)} + 1.5 \right\rfloor \\ 31 &\approx \frac{253 \ln(3.858)}{\ln(r_\max)} \\ \ln(r_\max) &\approx \frac{253\ln(3.858)}{31} \\ \ln(r_\max) &\approx 11.02 \\ r_\max &\approx 61020 \end{align} $$

Since most of the image is white, I would expect a large spike at the 0 frequency point with an amplitude near $NM = 256^2 = 65536$ (where $N$ and $M$ are the width and height of the image). So $r_\max \approx 61020$ is quite plausible.

So for this image (and this image only, since different images would have a different dynamic range), the approximate scaling would be:

$$ \begin{align} \mbox{value} &\approx \left\lfloor 22.96 \ln(\sqrt{X_\text{Re}^2 + X_\text{Im}^2}) + 1.5 \right\rfloor \\ &\approx \left\lfloor 11.48 \ln(X_\text{Re}^2 + X_\text{Im}^2) + 1.5 \right\rfloor \end{align} $$