5
$\begingroup$

To preface, this is not a homework related question but purely for self-study purposes.

If I am given the following Pole-Zero Plot: (Source: Berkeley Exam1)

  • How would I go about trying to determine whether the system is causal or stable?
  • Similarly, why should the region of convergence be $0.5 < |z| < 2 $ for stability?

I know that for stability, the ROC must include the unit circle. And for causality, $h[n]=0$ for $ n<0$. Other than that, I am having trouble applying those rules to a pole-zero plot.

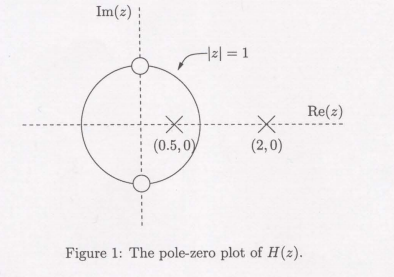
enter image description here

Improve this question
$\endgroup$

1 Answer 1

Reset to default
5
$\begingroup$

For systems with more than one pole (with different radii), there are $3$ types of ROCs:

  1. inside the circle with a radius corresponding to the smallest pole radius; the corresponding time-domain sequence is left-sided

  2. outside the circle with a radius corresponding to the largest pole radius; the corresponding time-domain sequence is right-sided

  3. in a ring defined by the radii $r_1$ and $r_2$ of any two poles with $r_1<r_2$, with no other pole at a radius in the interval $(r_1,r_2)$; the corresponding time-domain sequence is two-sided

In your example you have case $3$ above, because that's the only ROC that includes the unit circle, and, consequently, corresponds to a stable system. Both other possible ROCs (inside the circle with radius $0.5$ and outside the circle with radius $2$) do not include the unit circle, and, consequently, do not correspond to impulse responses of stable systems. Since a ROC equal to a ring between two poles corresponds to a two-sided sequence, the system can't be causal. A causal system has a ROC outside a circle (case $2$ in the list above).

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.