Measuring the open loop transfer function in closed loop: what's the better approach?

Question

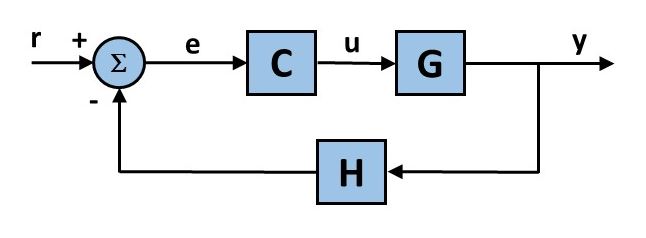
Considering the closed loop system; $C$,$G$, and $H$ all linear and stable transfer functions,

• If I chose to excite $\bf r$ and measure $\bf e$, I get the Sensitivity Function, $S$ $$S=\frac{1}{1+CGH}$$

• If I however excite $\bf r$ and measure $\bf y$, I get the Complimentary Sensitivity Function, $T$

$$T=\frac{CGH}{1+CGH}$$

• If $G$ or $C$ contains integrators then $S$ tends to be high pass and $T$ low pass.

So my question: In terms of signal to noise ratio on $\bf r$ and $\bf y$, what is the better choice in determining $G$ from the closed loop measurements?

$$G=\frac{1-S}{SCH} \quad \text{or}\quad G=\frac{T}{(1-T)CH}$$

It seems in one situation information is attenuated at low frequency and in another at high frequency. So for practical purposes would one method have advantage over another depending on whether noise dominates the input or output signals measured?

Answer 1

You have two signals to use, the reference and the measurements. You generate the error from these two signals. There is no additional information in that signal.

Closed-loop identification is more difficult than open-loop because the feedback makes the input and the noise correlated. Consider the standard feedback system

where $\bar{C} = C H$, $G_{0} = G$, $r_{2} = 0$, $r_{1} = C r$ to make it equivalent to your block diagram. A direct identification of a model $G_{m}$ means minimizing $$ V(\theta) = \frac{1}{2\pi} \int_{-\pi}^{\pi} \left| G_{0}(\text{e}^{j\omega}) - B(\text{e}^{j\omega}) - G_{m}(\text{e}^{j\omega},\theta) \right|^{2} \frac{\Phi_{u}(\omega)}{\left|H^{*}(\text{e}^{j\omega})\right|^{2}} $$ where the bias term is given by $$ B = \frac{\bar{C} S_{0} H_{0} \Delta H {\sigma_{\bar{e}}}^{2}}{\Phi_{u}} $$ and $\Delta H = H_{0} - H^{*}$, where $H^{*}$ is an assumed, fixed noise model, and $S_{0}$ is the input sensitivity function of the diagram. The bias in the model estimate $G_{m}$ will be small if

• The noise model $H^{*}$ is accurate.
• The signal-to-noise ratio at the input $u$ is large.

The estimation can be improved by using more elaborate identification methods. A great survey is found in Chapter 9 here.

The choice of excitation signal in order to minimize your estimation variance is discussed in Chapter 7 in the above reference, and another great paper can be found here.