Minimum Energy Delay Property

Question

I have 4 systems which are represented in z domain like this:

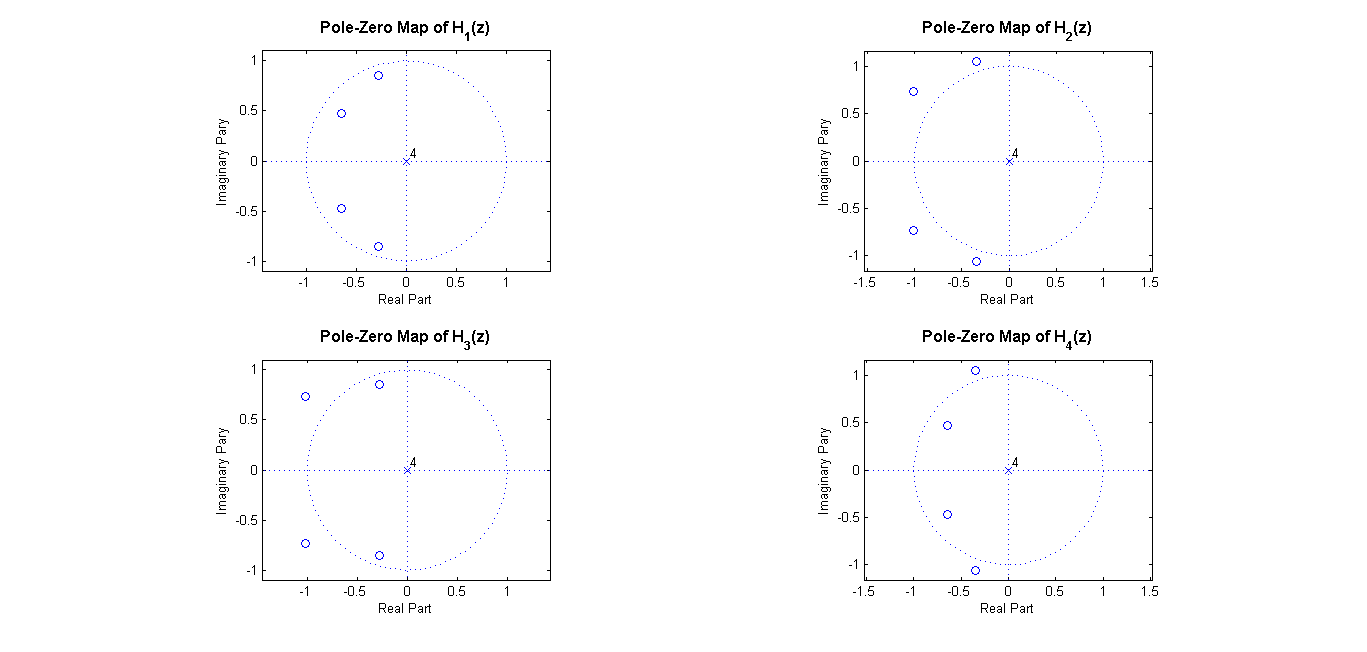
Where I know the poles and zeros.

Then I compute Partial Energy Sequence of impulse response of all these system using this formula:

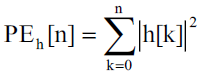
And I get this result:

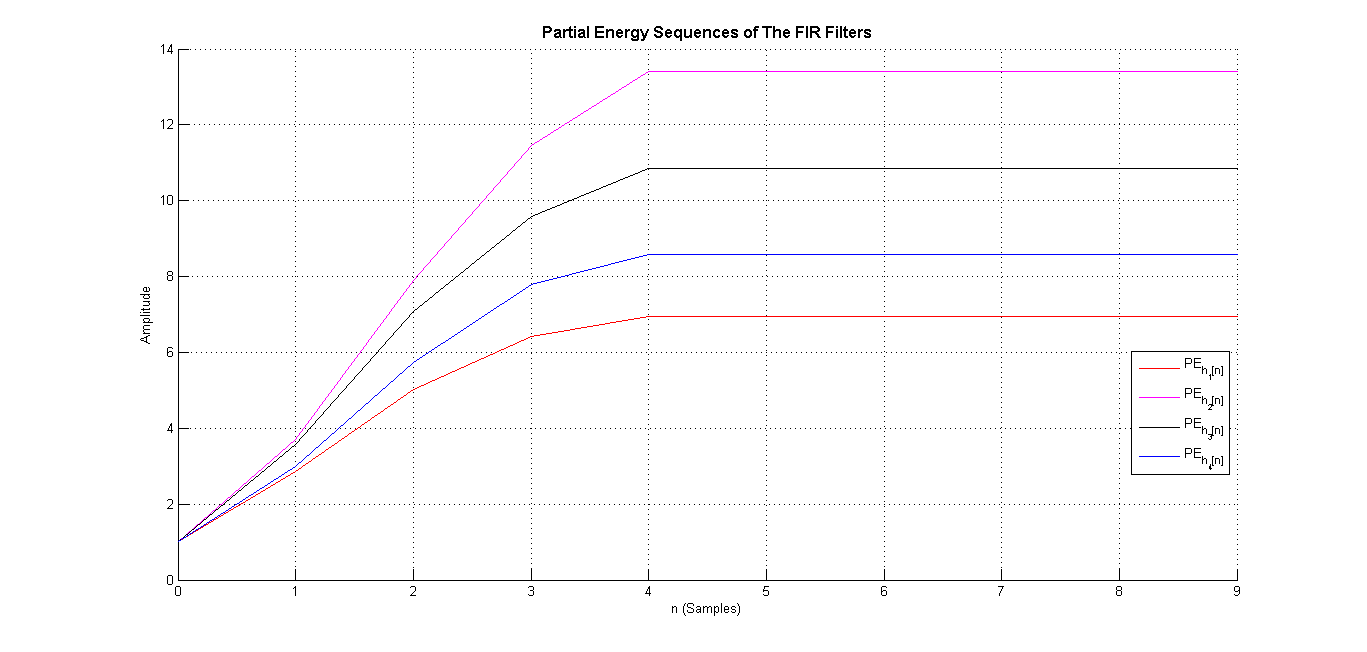
And I want to observe minimum energy delay property of the minimum phase systems can be specified as:

If I am right, the formula above tells us that minimum phase system has a bigger or equal at least partial energy but, unfortunately, you can see in partial energy sequence plot, the minimum phase system has the smallest partial energy!

Am I interpreting the minimum energy delay formula wrong or what am I missing here?

Answer 1

Given only the zeros and poles, the impulse response is determined except for a constant $K_i$, which you don't show.

From your calculations, it is clear that the energy of the impulse response for all filters is different.

If you normalize all filters so that all impulse responses have the same energy (i.e. $\sum_n |h_i[n]|^2$ is the same for the 4 filters), then the 4 curves for partial energies will end up at the same value, and it will be clear that $H_1$ has its energy distributed earlier in time than the others.