- Can't capture high $f$: insufficient sampling rate.
- Can't capture low $f$: insufficient observation interval.
The DFT is a machine that spits out frequency measures, done by correlating against finite bases. If $x(t)$ is some frequency, its DFT will peak at that frequency, meaning the inner product against a whole number of cycles is maximized. But who says the number of cycles must be whole?
Nothing, nor does anything say that a whole cycle is sufficient: for DFT at 100Hz sampling rate lasting 2 seconds, "enough" for a 1Hz signal is 2 cycles, and for a 10Hz signal it's 20 cycles, yet from a physical standpoint, there's no such x10 difference in requirement. Whole cycles do provide nice properties, but don't change legitimacy of our estimate.
Measuring "sufficiency"
Fundamentally, it's a problem of information scarcity, or time-frequency resolution. This is readily seen in the Continuous Wavelet Transform: the lowest frequency coefficients are of worst quality, which we attribute to "boundary effects", i.e. operating outside of known signal. It's not discussed much, but CWT is actually the one with fixed resolution, not STFT, in arguably the more meaningful sense: a picture of 4Hz and 2Hz, and a picture of 8Hz and 4Hz, look same if each case zooms to the higher frequency's period, like an apple twice as far appearing half as large:

which is captured directly in the CWT basis:
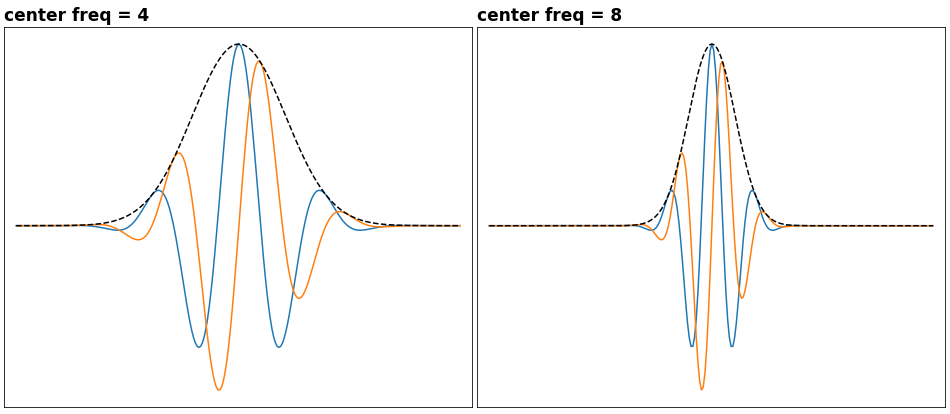
Note, doubled frequency has doubled number of oscillations per unit time, but same number of oscillations under the envelope, i.e. per observation interval.
So, how long an observation is long enough? Suppose we know what it is. Say, to fully capture a 2 meter tree, hold camera from 5 meters away. Then, to fully capture a 4 meter tree, hold camera from 10 meters away. By same logic, if we declare 10 cycles is good enough for 2Hz, then 20 cycles is good enough for 4Hz. Then, how far we are off from "good enough" will appear in CWT's boundary effects region (image from MATLAB):

where right at bottom, nothing is good enough!
So, it's a matter of statistics: window size in relation to frequency provides a measure of our confidence. Luckily, resolution measures have statistical mathematical equivalents.
Now, a part of the CWT argument is a prior. An assumption on the model to best fit reality. It's possible STFT is a better fit for a given task, e.g. if (completely making up) higher energy worsens particle localization hence a higher frequency photon requires a greater observation window. What holds either way is, this is a matter of information, well-handled by statistics and heuristics.
Lastly, a correct but incomplete point is, insufficient SR = can't recover perfectly, but insufficient window can recover. Problem is, the insufficient window must have a sufficient SR - what this actually requires, though, is for the window to be long enough! That's because "sufficient SR" presumes "bandlimited", which is only satisfied with an infinite observation window, or window that captures at least one full period of a periodic input.



