1
$\begingroup$

I was reading this and it explains the various windows such as Rectangular, Bartlett, Hanning, Hamming, and Blackman. Specifically, I saw this graph comparison of their dB magnitude frequency response for M=50. 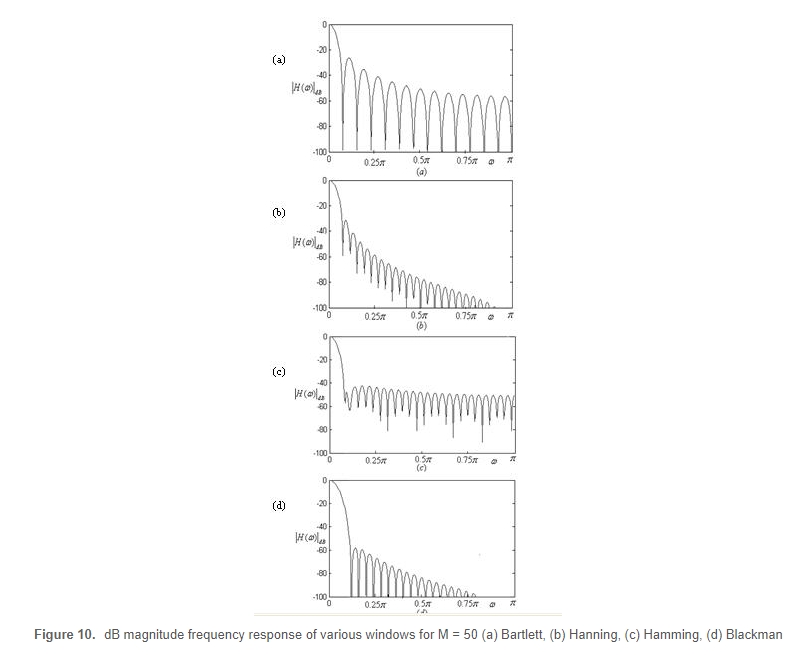Window Techniques

Obviously, there are differences between the techniques. However, as a newbie in the subject, I can't quite put it into words. I know it involves their passband ripple, stopband attenuation, and transition regions, but further than that I can't put out into words what are the differences of these window techniques in terms of the parameters mentioned above.

[EDIT] I need the appropriate technical adjectives for the parameters. For example, am I right in saying that the passband ripple gets larger from (a) to (d) because the magnitude gets steeper at the start of the graph from the left? And for the stopband attenuation, it gets smaller from (a) to (d) because the magnitude of the bounce as it gets to the right of the graph gets smaller? For the transition regions, it gets smaller and the transition gets finer (?) from (a) to (d) since the width of the bounces gets smaller?

[EDIT] The words I use doesn't seem right, or not that appropriate. I feel like there could be better adjectives to describe their differences in passband, stopband, and transition.

Can someone please help me compare these techniques into words? Thank you very much!

Improve this question
$\endgroup$
9
  • $\begingroup$ It seems you have all the words I would have used! $\endgroup$
    – Marcus Müller
    Commented Jun 8, 2021 at 9:03
  • $\begingroup$ I don't really know what you're asking here: you know the applicable terms, so apply them. For example, you could just take (a) and (c) and compare them according to these terms, and add that to your question. Then ask about something that's been unclear to you, specifically! It's really not clear what your question is right now, sadly. $\endgroup$
    – Marcus Müller
    Commented Jun 8, 2021 at 9:10
  • 1
    $\begingroup$ @MarcusMüller hi! Thanks for the reply. I further edited my post, although this is really the extent of what I can observe and I need help in correcting my, as you put it kind of correctly, guesses. From my understanding, the passband ripple is the leftmost jump downward. As it goes from graphs (a) to (d), the magnitude $$H( \omega )$$ of the passband ripple seems to get larger, that's why I "guessed" it gets larger. (1/2) $\endgroup$
    – John Smith
    Commented Jun 8, 2021 at 11:44
  • 1
    $\begingroup$ ...(2/2) For the stopband attenuation, from my understanding, it is the magnitude $$H( \omega )$$ of the 'bounces', that 's why I also said it gets smaller because the $$H( \omega )$$ tends to go downwards from (a) to (d), like in (d) the bounces are not even in the frame anymore. For the transition, from my understanding, it is the width between the bounces itself. So I said the transitions seems smaller and finer because the bounces looks more and more compacted from (a) to (d). But as you kind of have caught me, these really are just guesses, and I need help correcting my guesses. Thanks! $\endgroup$
    – John Smith
    Commented Jun 8, 2021 at 11:47
  • 2
    $\begingroup$ Please find a copy of fred harris' classic paper "On the Use of Windows for Harmonic Analysis With the Discrete Fourier Transform". It is a wonderful paper that details and compares all of the key window parameters including terminology. $\endgroup$
    – Dan Boschen
    Commented Jun 9, 2021 at 4:40

1 Answer 1

Reset to default
2
$\begingroup$

In this case the Wikipedia article is quite helpful: https://en.wikipedia.org/wiki/Window_function

In general you are looking at different properties and here are some common names for these:

  1. Width of the main lobe.
  2. Location of the first side lobe
  3. Height of the first (or biggest) side lobe
  4. "Average" stop band attenuation, where the exact method of averaging and definition of "stop band" depends on your application

Another term that's occasionally used is "scalloping loss" "https://www.recordingblogs.com/wiki/scalloping-loss

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.