Ideal sampling - question about the 1/T scaling factor

Question

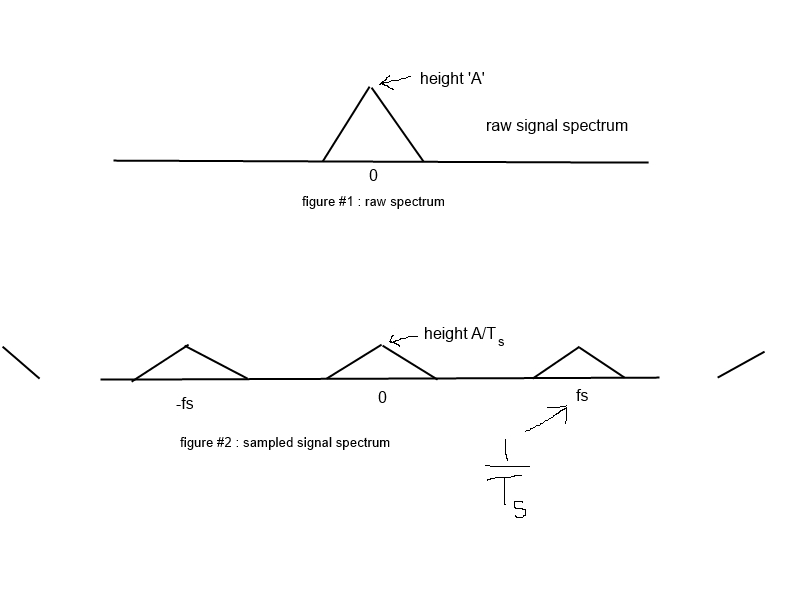
Sources discussing spectrum of sampled signals (under 'hypothetical' IDEAL SAMPLING condition) show that the original message spectrum gets replicated at integer multiples of the sampling frequency.

It's noticed that the sources show that the replica spectra (seen in the sampled spectrum) are scaled by a factor 1/Ts .... or 1/T (where T aka Ts is the sampling period, and 1/Ts is the sampling frequency).

My question I'd like to ask is --- it appears that when the sampling period gets relatively small (or relatively tiny), which means sampling frequency gets relatively large, then the scaling factor 1/T becomes relatively large.

So if the sampling period 'approaches' zero, then the 1/T scaling 'approaches' an infinite value, right? In that case, will each of those peaks of the replica spectra (in the spectrum of the sampled signal) become infinite?

I'm not yet able to understand the implications of the factor 1/T for the case where the sampling period becomes really tiny. It just seems to me that the spectral peaks work towards becoming infinite as T gets mathematically reduced toward zero. Have I understood this correctly? Are the spectral peaks meant to become larger and larger with reduced sampling period (aka larger sampling frequency)? I don't understand why the amplitudes can become really large if T is really small. Will this lead to an infinite power or infinite energy condition?

The main thing I'm currently confused about is - if the peak value of the original signal spectrum is 'A', then the peak value of the sampled signal spectrum is A/T (ie. A/Ts), but since 1/Ts is typically a finite number much greater than 1, then the peak value of A/Ts could be some pretty big number. It's as if there's some kind of huge amplification factor introduced due to 'ideal sampling'. Are we meant to just accept this 'big' 1/T amplification factor?

Thanks all!

Answer 1

You sound right.

The impulse modulation description of ideal sampling operation introduces an amplitude scale of $1/T$ on the spectrum of the sampled signal, which is a periodic replica of the original spectrum.

Note that as the sampling period $T$ gets shorter, the scale factor gets larger and the separation between repeating replicas gets wider too.

This is an ideal operation. In practice, what you are interested in is the finite valued samples of the signal at the sampling instants; the sample values (envelope) will be the same irrespective of the sampling period.