Nyquist Stability Test

Question

Currently I'm learning about Nyquist Stability Test and I'm few days stuck on one thing and I don't understand that so I wanted look here for help.

Given is transfer function $K\frac{2s-11}{s(s^2+s+1}$. We take that $K=1$.

Line represens the Nyquist contour for K=1.

Than in my book it's written that that function is stabil when

$\triangle arg(1+L(Iw))=(max(grad(z_L),grad(n_L)-N_{-}(n_L)+N_{+}(n_L) )\pi$

than they see from this picture above $\triangle arg(1+L(Iw))=-\pi$

and

$(max(grad(z_L),grad(n_L)-N_{-}(n_L)+N_{+}(n_L) )\pi=(max(1,3)-2+0)\pi=\pi$

Not stabil.

What I don't undestand is - $\triangle arg(1+L(Iw))=-\pi$? What this could be? What angle? How do I see that from picture?

Answer 1

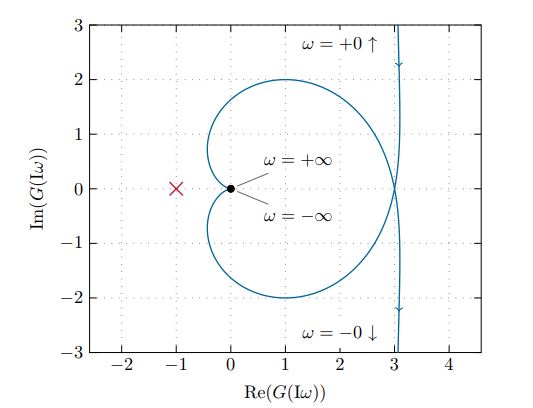
The open-loop transfer function

$$G(s)=\frac{2s-11}{s(s^2+s+1)}\tag{1}$$

given in your question has the following Nyquist plot:

which is different from the one in your question.

Note that in any case it's important to know how the trace is closed, i.e., how the points $\omega=0^-$ and $\omega=0^+$ are connected. In this case they are connected by a semi-circle with infinite radius in the left half-plane (the dashed curve in the figure below):

[generated with WolframAlpha]. This is a consequence of the pole of $G(s)$ at $s=0$.

The Nyquist criterion is actually simpler than you made it look in your question. The number of clockwise encirclements ($N$) of the point $-1+j0$ equals the number of right half-plane zeros of $1+G(s)$ ($Z$) minus the number of right half-plane poles ($P$):

$$N=Z-P\tag{2}$$

Since $G(s)$ has no poles in the right half-plane ($P=0$), the number of clockwise encirclements equals the number of zeros in the right half-plane. From the Nyquist plot we see that $N=1$, so there is one zero in the right half-plane, which corresponds to one pole of the closed-loop transfer function in the right half-plane. Consequently, the system is not stable.

This is also easily verified by directly computing the poles of the closed-loop transfer function

$$Q(s)=\frac{G(s)}{1+G(s)}=\frac{2s-11}{s^3+s^2+3s-11}\tag{3}$$

roots([1,1,3,-11])
ans =

  -1.2833 + 2.3182i
  -1.2833 - 2.3182i
   1.5667 + 0.0000i

which shows that there is one pole with a positive real part, as predicted from the Nyquist plot.