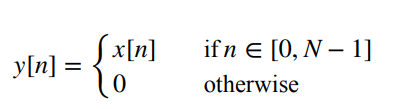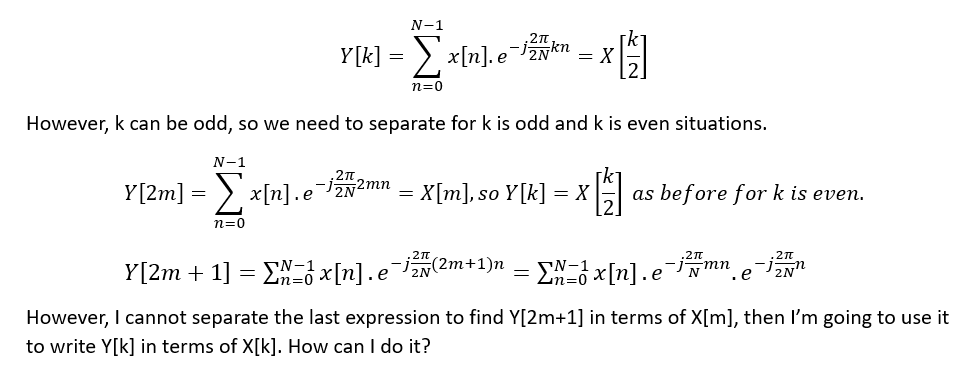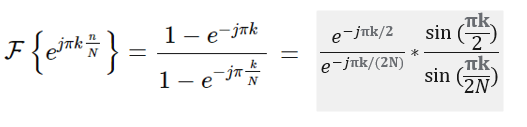$N$ is an even integer, $x[n]$ is a finite length signal over the interval $n \in [0,N-1]$, and $X[k]$ is the $N$-point DFT of $x[n]$. Analytically find the DFT of sequence below in terms of $X[k]$. DFT size is $2N$ and
So, I did this (note: below, in $Y[k]=X[k/2]$, $k$'s being odd creates indefiniteness.)
I found the exponential terms in terms of sine appears on both nominator and denominator.