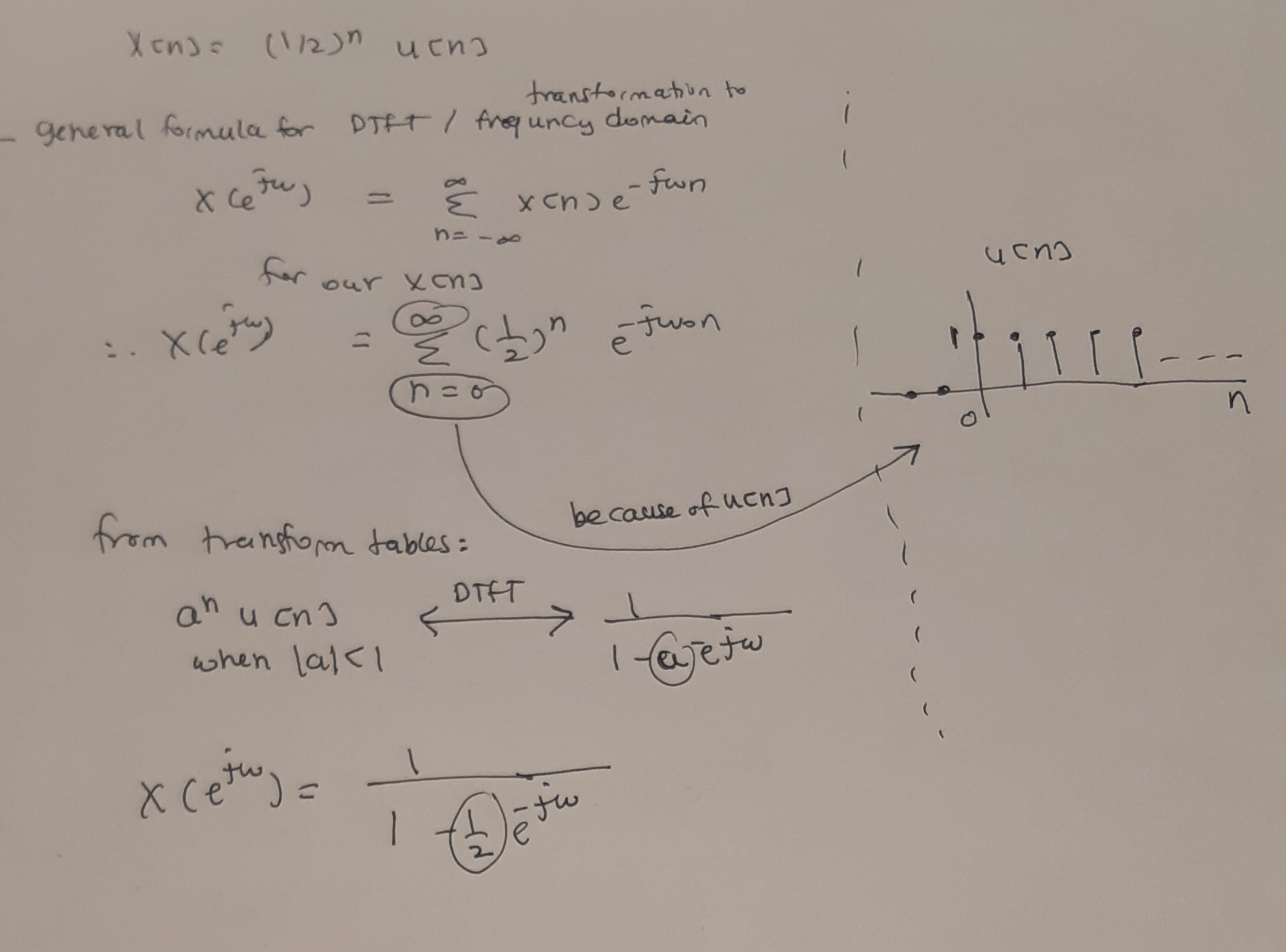
When calculating DTFT of $\left( 1/2 \right)^{n} u \left[ n \right]$. We evaluate the sum as follows:
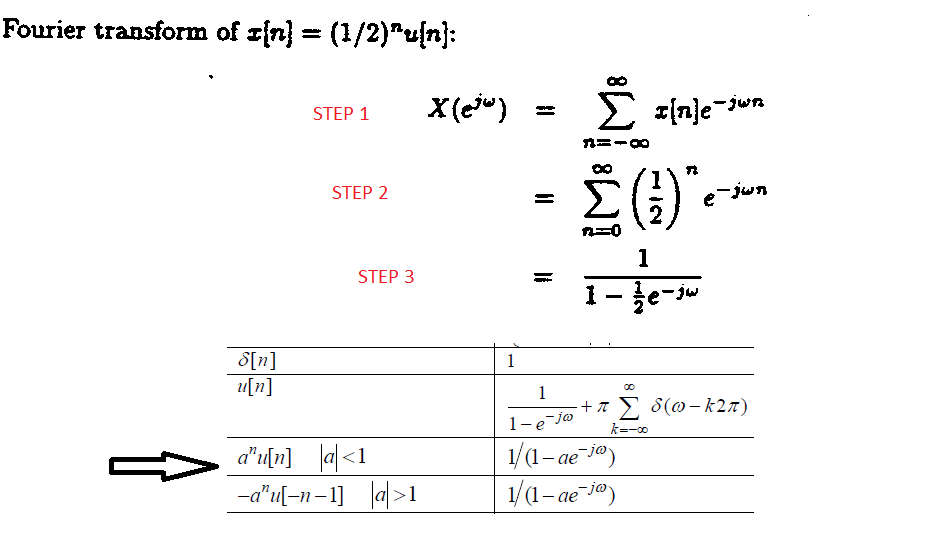
Please correct statements and answer questions below:
So to go from STEP 1 to STEP 2, the limits of the series are changed from $-\infty$, $+\infty$ to 0, $+\infty$ because $u \left[ n \right]$ is $1$ for $n \geq 0$ and $0$ otherwise. Correct?
In STEP 2 we substitute the value of $x \left[ n \right]$ so the term $u \left[ n \right]$ is not found in STEP 2 because it is evaluated as $1$. Correct?
If term $u \left[ n \right]$ is no longer in STEP 2 equation how can we use the transform equation (indicated by arrow) in table when only $ \left( 1/2 \right)^{2}$ remains?
If you have a different explanation on how to transition from STEP 1 to STEP 3. Please feel free to comment.
My solution is below. I want to comment but I cannot attach images in comments.