To answer this you need to understand what is a pole and what is a zero of a transfer function.
Let's look at a simple 2 poles 2 zeros filter (also called biquad filter) transfer function : $$ H(z) = \frac{b_0+b_1 z^{-1}+b_2 z^{-2}}{1+a_1 z^-1 +a_2 z^{-2}} $$
This can be factored as : $$\begin{align} H(z) &= \frac{ b_0 \, (1-q_1 z^{-1})(1-q_2 z^{-1})}{(1-p_1 z^{-1})(1-p_2 z^{-1})} \\ \\ &= \frac{ b_0 \, (z-q_1)(z-q_2)}{(z-p_1)(z-p_2)} \\ \end{align}$$
Where :
- $p_1, p_2$ are the poles of the filter ;
- $q_1,q_2$ are the zeros of the filter.
When we evaluate frequency response of a discrete-time filter, we evaluate the transfer function with $z = e^{j\omega}$. The magnitude of $H(e^{j\omega})$ or
$$\begin{align} \Big|H(e^{j\omega})\Big| &= \left| \frac{ b_0 \, (e^{j\omega}-q_1)(e^{j\omega}-q_2)}{(e^{j\omega}-p_1)(e^{j\omega}-p_2)} \right| \\ \\ &= |b_0| \frac{|e^{j\omega}-q_1| \, |e^{j\omega}-q_2|}{|e^{j\omega}-p_1| \, |e^{j\omega}-p_2|} \\ \end{align}$$
is the quantity of interest here. (The phase of $H(e^{j\omega})$ is perhaps of interest, but is not about the topic asked.)
$|b_0|$ is a constant. $|e^{j\omega}-q_1|$ is the distance between a point on the unit circle $e^{j\omega}$ and the zero $q_1$. $|e^{j\omega}-p_1|$ is the distance between a point on the unit circle $e^{j\omega}$ and the pole $p_1$. Note that in the frequency response magnitude, we are multiplying by the distance to the zeros and dividing by the distance to the poles. Multiplying by smaller numbers makes your result smaller. Dividing by smaller numbers makes your result larger.
Now simple maths tells you that if $z$ approaches $p_1$ or $p_2$, the denominator will approach $0$ and thus the whole transfer function will approach a gain of $\infty$ (as $\frac{A}{0}\rightarrow \infty$). On the other hand, if z approaches $q_1$ or $q_2$, the numerator will approach $0$ and the whole transfer function will appraoch a gain of $0$ (as $\frac{0}{A}\rightarrow 0$).
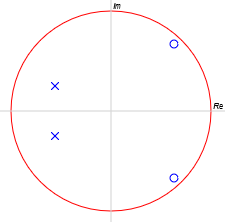
Another way to look at it is with the complex circle:
The poles $p_1, p_2$ are on the left, the zeros $q_1, q_2$ are on the right. The point $e^{j \omega}$ is some point on the unit circle (in red).
On the right side of the unit circle is yourwhere $\omega=0$ at $\Re\{z\}=1, \Im\{z\}=0$$z=e^{j0}=1+j0$, which is the point at DC. On the left side isof the unit circle where $\omega=\pi$ at $\Re\{z\}=-1, \Im\{z\}=0$$z=e^{j\pi}=-1+j0$, which is the point at the Nyquist frequency. As you go through the frequency response starting at DC, you "walk" around the unit circle, counter-clockwise.
The closer you get to a pole, the higher the gain of the filter is because you are dividing by a smaller number. The closer you get to a zero, the lower the gain because you are multiplying by a smaller number.
It's as simple as that!
You might want to play around with this applet, it's a good illustration.
Good luck for your exam!