@MathBgu I have read all above given answers, all are very informative one thing iI want to add for your better understanding, by considering the formula of convolution as follows 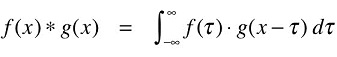
$$f(x)*g(x)=\int\limits_{-\infty}^{\infty}f(\tau)g(x-\tau)\,d\tau$$
and for the cross correlation
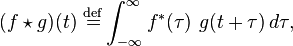 $$(f\star g)(t)\stackrel{\text{def}}{=}\int\limits_{-\infty}^{\infty}f^*(\tau)g(t+\tau)\,d\tau,$$
$$(f\star g)(t)\stackrel{\text{def}}{=}\int\limits_{-\infty}^{\infty}f^*(\tau)g(t+\tau)\,d\tau,$$
we comescome to know that equation-wise the only difference is that, in convolution, before doing sliding dot product we flip the signal across y-axis i.e we changeschange (t)$(t)$ to (-t) $(-t)$, while the cross correlation is just the sliding dot product of two signals.
We use the convolution to get output/result of a system which have two blocks/signals and they are directly next to each other (in series) in the time domain.
